Applicability of RT60 and measurement thereof to "small acoustic spaces."

[amirm]

In acoustics, home listening spaces are considered to be small. Some people take that and then use it to invalidate some of the classic measurements such as RT60 which shows how long reflections take to die down in a room. I have argued this point elsewhere with a lot of detail, showing that if we know what we are doing, we can measure and trust RT60. Ethan was the subject of one of those discussions and he had the good idea of distilling it to a short article. I thought it would be a good read for folks here:

http://www.realtraps.com/art_reverb.htm

I will also post copies of my posts from other forums on this topic.

 

[amirm]

To state my point more clearly, I'll quote from the same book in Chapter 8, Small Room Acoustics:
My point (to clarify) is not to prove one wrong about the reverb definition (I simply don't care the term one uses, I use the terms interchangeably depending on who I'm talking to), but to simply state: the RT60 calculation is useless in a small room.

You have not given us any independent data why you believe that. So let's go to the horse's mouth and examine how strong Davis' proof points are that such a measurement is "meaningless" as he states.

The section you are quoting is less than a page long and is titled, “Small Room Reverberation Times”. I will address the theory points he puts forward in another reply but for now, let’s examine the back up he puts forward in the form of a quote from Dr. Schultz’s 1963 AES Journal paper, ”Problems in the Measurement of Reverberation Time.” Unfortunately Davis' only shows you a snippet instead of the full context of that (very good) paper. I will remedy that here.

As the title indicates, the paper is about what can go wrong in measuring reverberation times using various techniques. He starts with this case study:

Consider the curves shown in Fig. I, which purport to display the reverberation time of a small rectangular room plotted as a function of frequency. The upper curve gives the reverberation time when the room is completely bare. It shows an extreme variation of reverberation time with frequency. The lower curves were obtained by adding progressively more sound absorptive material to the room.”

“CPS” is cycles per second or as we commonly call it, Hertz. So the chart’s horizontal axis ranges from 10 Hz to 10,000 Hz. He goes on to explain what is wrong:

”What, then, is the trouble with these curves? Surprisingly, there appear to be at least three methods of measurement which would give this kind of wrong answer! The first of these consists of measuring the reverberation in the room indirectly by means of a standard acoustical source of known power output. This method works fairly well in a large room in which a great number of the room modes crowd together and overlap in any reasonably small frequency band.”

So the key to the measurement being correct is having many modes overlapping. Where do we run afoul of this in our small rooms? He explains it:

“In a large room, if one has a large sound source whose power output is known, one can determine the total amount of absorption in the room by measuring the average pressure throughout the room. This total absorption can then be used to calculate the reverberation time from the Sabine formula. This method fails badly in a small room, however where, a large part of the spectrum of interest lies in a frequency range where the resonant modes of the room do not overlap but may be isolated , as shown in Fig. 1 (individual resonance peaks are identified with their corresponding mode numbers). In this case the microphone, instead of responding as a random sound field (as required for the validity of the theory on which these methods depend), will delineate a transfer function of the room. This is a curve which gives response in terms of modal frequencies, but only a little about the absorption on the boundaries. It does not provide a valid measurement for the reverberation time in the room however.”

The above is the only part of the paper quoted in Davis’ book except that he omits the sections in bold. When we add them in and focus on key sections I have highlighted in red, we realize that this is not at all supportive of the broad assertion made by Davis and you repeating the same. Dr. Schultz is focused on the modal region below 100 to 200 Hz and saying that we do not have many modes in small rooms so we better be careful in applying simple RT60 formulas.

What does this mean in English? Modes create peaks and dips in the room response at certain frequencies which we can theoretically predict using modeling. Let’s use Ethan’s tool and apply it to a typical home listening space which in acoustic domain is considered “small:”

We see that at frequencies less than 100 Hz, the modes are indeed pretty sparse. To wit, below 50 Hz with have just one at ~28 Hz. Let’s compare this to a much larger room:

Quite a different picture emerges as we now get lots of room modes in the same low frequency area below 100-200 Hz. I want to reserve the theory of why we need many modes for the next post but for now, let’s accept what we want is what Dr. Schultz says: many modes for the reverberation times to be more accurate.

One “solution” to getting more modes is to do per above which is to have a much larger room. But there is another: move up in frequencies. Look at the density of modes on the right edge of our small room: it is starting to have lots of modes packed together much like what the larger room has at lower frequencies. Translating, if the RT60 measurements are accurate to a low frequency in large room, they are accurate just the same for small room except that we need to move up in frequencies.

If you search for my posts and countless peer reviewed literature in Journal of ASA and AES which use RT60 measurements in small spaces, the above is precisely the recommended practicethey do: the RT60 measurements are for “mid-frequencies.” I use 500 to 1000 Hz; others use the same or go up to 3 KHz or so. RT60 is not provided as a measure to evaluate modal (e.g. subwoofer) region. There we use frequency response to reveal the impact of the modes. Therefore, our practice and that of countless other researchers is very much in compliance with the concern that Dr. Schultz shares.

So the claim of the measurement being useless is not supported by the citation in Davis’ paper. He creates a scenario (using it in modal frequencies) and then shoots it down.

To be fair, at mid frequencies our modal distribution in a small room has not yet fully achieved Poisson distribution (discretely random in space and time but with a known long term average). I will address why this is not an impediment to analysis of our small spaces in the next post.

As if he knows that the Dr. Schultz point only applies to modal low frequency region, Davis’ next immediate section, 8.4 titled “Small Room Resonances” is a discussion of room modes!

Believe it or not the best part of this answer is yet to come! In this section, he provides this graph:

Seems familiar, no? It is the exact same measurement as Dr. Schultz used sans the repeat sin of saying where it came from! This is the text that goes with that graph:

”In Fig. 8-4, the effect of “undamped” modes are plotted, as a decay time, for small broadcast studio. The damping is provided by diaphragmatic absorption at the lower frequencies. “

Get it? He uses the very graph Dr. Schultz used to say represents wrong information, renames it to “decay time” and claims it now holds valid data!!! How could a measurement go from meaningless to so useful by just changing the term that way? Note how the graph still says Reverberation time!

It gets more interesting. Dr. Schultz’s paper was published in 1963. The measurement above must have been performed prior to 1963 or else, Dr. Schultz could not have quoted it. The first revision of Don Davis’s book which by the way did not have professor Patronis involved came out in 1987. How is it that someone writing a text on acoustics has nothing more current and appropriate to use to demonstrate room modes than a measurement from some 25 years back??? And one that completely torpedoes his case just half a page back??? Your guess is as good as mine but I am afraid it indicates that maybe Davis did not read the Dr. Schultz paper either.

I don’t want to take anything away from Don Davis’ reputation or work. But let’s all agree that this is not how we go backing strong assertions. Take a look at the Dr. Toole coverage of the same topic and it goes on for tens of pages, encompassing research paper and data after research paper and more data. You can go read any of the original research as I have done and you will have a heck of a hard time invalidating the conclusions he draws from them. Everything in Dr. Toole’s case is referenced properly so that people can double check if they want. Not so here sadly.

Summary
Davis makes a very bold claim that RT60 measurement is meaningless. Folks proceed to repeat the same all of the Internet and hammer into people as gospel. Yet just one level of digging shows that the backup data Davis uses refers to not using RT60 for low frequency analysis. That is a non-point since the proper use of RT60 is for analysis of mid-frequencies, not modal low frequencies. Worse yet, Davis turns around uses what is said to be faulty data as his proof point for something else by just swapping names! I get believing in what experts tell us but come on now. People in the industry should at least dig one level further and read research references before believing.

As I noted in part two of this post (in the next few days) I will address the theory points Davis’ sites for those who really want to know “how the sausage is made.”

 

[amirm]

Nice post Amir. I suspect it's falling on deaf ears.

Thanks Ethan. Even though you are very much right, I think it is worthwhile to set the record straight with respect to the data/science being put forward. On that front, as you recall we had this same discussion about a year ago. I am sure you remember this poster who Local copies in both style and substance giving you the same as arguments as him but with this additional specificity (underline mine):

Manfred Schroeder defined the large room frequency, and derivatively, the volume of a room necessary to support a reverberant field at the large room frequency and above.

There is NO reverberant sound field below that frequency. Since we are concerned with volume, and using a generally accepted breakpoint value for RT of 1.6 seconds:

Volume = K^2 (RT60/(Lg Room freq^2)) K in SI (metric) is 2000 and 11,885 in imperial/US terms

Thus, for 300 Hz and above, the room must be a MINIMUM of : (11,885)^2 (1.6/(300^2)) = 2511.168 or 2511 ft^2

When someone has a room larger than 2511 ft^2 and is ONLY concerned with reverberant times ABOVE 300 Hz, determined properly - NOT with a directional home speaker but with a true omni-directional source stimulus - call me.

Let's forgive the typo there where he says the volume is feet squared rather than cubed and perform the same math for two rooms, the one that I mentioned in my last response and your garage:

My "small" listening room was 19 by 12 feet and had a height of 8 feet. That makes the volume 1,824 feet^3. Computing the transition frequency as the above number is called, we get 352 Hz.

For your garage, I am guessing that that it is roughly 30 by 20 with a 10 foot ceiling. That gives us a volume of 6,000 feet^3 resulting in a transition frequency of 194 Hz.

Recall how this argument started with Local stating, "again, to limit confusion to other novices on this forum, there is no appreciable reverberant sound-field at any frequency us humans are concerned with in small acoustical spaces (residential rooms, living rooms, etc)."

I think we all agree that humans are very much concerned with frequencies above 194 to 352 Hz in the above example spaces. We have the rest of the response all the way up to 20,000 limit of our normal hearing. So therefore Local’s statement is not supported by the evidence his mentor puts forward.

Local also said, "if you are challenging this notion then you are challenging the entire careers of Leo Berenek, Manfred Schroeder..." Seeing how the formula above came from Schroeder, he can rest in peace knowing we are not abusing his findings .

To be fair, there was a condition of "omni-directional" source put forward. In your garage you were making noises. I would say those noise sources are fairly omni-directional. You were in the middle of a large space making sounds in mid-air. So we now essentially satisfy both conditions as stated by them for you to have a reverberant space above transition frequencies!

How about my room then? Speakers as a rule are not omni-directional at higher frequencies and we usually don’t hang them in the middle of the room. So perhaps that is their get out of jail card? I will have a lot more to say later about this when I dig deep into the theory of how we compute Reverberation time and its history but for now, let's look at a frequency response graph of a sample home (and therefore 'small') listening space:

You can easily see that the room has two completely different characters. On the left not only do we have large swings in frequency response, but they also vary based on what seat we measure at. Our response therefore changes with “space” (measuring/hearing location) and hence violates the precondition of a reverberant room being space invariant in its response as I mentioned in my last post.

Above transition frequencies of a few hundred hertz however, that problem essentially goes away. Seat to seat variations almost entirely go away and the response becomes very smooth – one that can actually be computed using the anechoic chamber response of the speaker! If that can be done, then the room impact is essentially non-existent (in this context). Therefore we have met the conditions of reverberant space even though our speaker was not omni-directional.

Of course there is some accuracy error at the extreme. But such an error also exists in larger spaces. You simply need to know what you are doing and in this case, it means being above the transition frequencies. Once there, for the purposes that we want to use this measure, namely, how much overall absorption we need in the room, this measurement generates sound data, pun intended.

So whether we stay with the evidence the other side puts forward or our own data, they all invalidate the strong claims made.

As I said, there is a lot more to come including bringing this theory down to real knowledge of how we (correctly) perform such measurements with tools like REW.

 

[amirm]

As promised, here is the deep dive into Reverberation Time. It is long but I hope that it tells the story from start to finish and hopefully provides useful information beyond settling this argument.

History Part I
The origin of Reverberation Time is from Wallace Clement Sabine who around 1890 performed a ton of experiments in his basement to see how long it would take for sound to decay 1,000,000 times once it is shut off. Expressed in dB, we get 60 dB which happens to also be the sound level of a human. Sabine discovered that the decay time was proportional to volume (V) and inversely so to the total amount of absorption (Sa): RT60 = K * V / Sa (K is just a constant)

That kind of makes sense, no? You have more reverberations in a larger space than small. And an empty room like Ethan’s garage has more of it than one stuffed with furniture.

The Sabine formula was a godsend for early acousticians. Remember, this is a century before we have personal computers and nearly as long before we had pocket calculators. Anyone could perform the above math by hand and immediately get a sense of how a room would “sound” even prior to building it. People then performed surveys of performance halls and realized there is good correlation between how they sounded for different applications and RT60. It is not often that complex physics of a room with sound waves bouncing and absorbing becomes this simple to analyze.

History Part II
Decades later, Schroeder advanced our understanding of room reflections by introducing the concept of transition frequency below which, the room resonances/modes are separate and hence not random. He came up with a formula to determine this frequency which I showed in my last post. In reality there is no single frequency delineation (nature hates sudden changes) but a range above which we have the randomness we desire. For our home listening spaces, that ranges from 200 to 300 Hz typically.

The second thing he did was to come up with a reliable way to measure reverberation time. This is called the Schroeder Integral and is simply the sum of the square of measured decaying sound. I will show how this works in a minute.

Measuring vs Predicting RT60
So far we have talked about computing RT60 using the Sabine formula. I call that the predicted value of RT60. Its simplicity means that one can find cases where the prediction does not work as well. Let’s take the simple case of having a fully reverberant room meaning it has diffused reflections from all the hard walls. Now put an absorber in it. By definition the absorber will not bounce back some of the sound wave energy hitting it. As soon as you did that, your room is no longer fully reverberant. Put another way, the idea of a reverberant one does not exist in a realistic situation in big or small rooms!

Now think of a practical situation. You have a room with some furnishings in it. You add an absorber to it. The furnishings in your room caused your space to not have fully diffused reflections. That means the absorber experiences a different situation than it did in the reverberation chamber above. Sabine’s formula will mispredict how much of an effect that product has since its absorption coefficient was determined in a more reflective situation.

Instead of using the simple formula we can actually measure the decay of reflections in the room. Once there, we are free of many of the limitations of Sabine RT60. This is one of the reasons I am pushing back on all the theoretical objections against RT60. Often the text cited is old and implied in there is that the issues raised relate to Sabine formula, not in situ version we use. Let’s see how this works.

Measuring RT60 with Room EQ Wizard (REW)
I am going to walk through how we can use REW to determine the proper value of RT60. If you have used REW, you know that it has a button for RT60. If you click on it, you may be puzzled that none of the graphs are named RT60. Instead, there are odd names such as EDT, T20, T30 and Topt. Let’s table that for a minute and learn a bit more about theory behind the measurement.

The concept for measuring reverberation is rather simple: excite the room with an impulse – a sharp spike of sound energy that has high/known amplitude and zero time – and then measure how long it takes for the reflections that are created as a result of it to die down by 60 dB. In practice we don’t use such impulses as they are hard to create in our rooms and can damage our gear. REW uses a modern technique called log swept sine which produces very good results without the need for loud test signals. But the end result is the same: we are measuring an “impulse” even though it sounds like a sine wave going from low to high frequency.

Let’s look at what happens when we hit the “Filtered IR” button. IR is short for Impulse Response (IR). This is what the output looks like:

The sharp peak at (roughly) zero time is our (computed) impulse. To the left we see our noise floor prior to our “impulse” playing. On the right we see us returning to the sane noise level after the reflections die off. Reverberations in a room drop off exponentially post the impulse going away. In the above graph the decay appears to be a straight line. The reason for that is that the vertical scale is in dB which is a log scale. Log is the inverse of an exponent so we wind up with a line.

Speaking of the line, there is one in black. That is the Schroeder integral. If this were an ideal reverberation chamber, we would have a perfectly straight line. We would then follow where it crosses the -60 dB point on the Y axis, and read our RT60 on the X axis which is in time. That is not the case here. Our line is not straight. We will fix this shortly.

Overlaid on the Schroder integral line is a blue line. If you look to the top right box, you see “Topt” selected. Other choices are T20 and T30. The latter two are standardized methods for finding the RT60 value from a less than perfect Schroeder integral. Instead of using the whole line to compute our RT60, we use a part of it and extrapolate from there. T20 uses the time it takes for reflections to die down between -5 and -25 (difference of 20 dB). Simply multiply that value by 3 and we get our RT60 time. T30 is similar and uses -5 to -35 and multiplies by 2. Both of these methods solve the problem of what to do if our noise floor is above -60 dB point. They also avoid looking at the start of the line which may be different. These are standardized computational methods per ISO 3382. As a result researchers use the same methods for consistency.

Topt is a much cleverer one in that it attempts to analyze the Integral and try to fit a line to it wherever it needs to start. Use Topt unless you have a reason not to (e.g. complying with ISO standard).

Looking at Topt line, we see that it pretty much tracks the Schroeder Integral except at the beginning of our impulse (between 0 and 50 milliseconds). There, we have a sharper drop than our Topt line represents and hence shorter reverberation time (the line would be steeper if we made it parallel to the early portion). That early part is called EDT (Early Decay Time). If you look at the box on the left, the RT time reflects that: Topt computation gives us an RT value of 0.659 seconds whereas EDT gives us 0.428.

What is the reason for that sharper early decline? The room that is being measured has carpeted floor. Once a sound wave hits that, it loses a lot of its power. Whatever bounces off the carpet is likely going to die soon. And once it does, it no longer contributes to the energy of the reflections that exist between the rest of the (reflective) surfaces such as drywall constructed walls. Another reason for the sharp drop is that in early stages we have strong reflections which push up the value of Schroeder Integral. Those go away after a while and we are left with our random/diffused reflections. As noted, all the standardized measurements of RT avoid the earlier part of the graph and hence, represent the “late” reflection timing of our room.

Back to our graph, REW provides a measure of how far off our RT measurement is from Schroeder integral with that “r” value (correlation coefficient). -1 is perfection meaning our single line matches the integral completely. Our Topt line is at -0.998 which is darn close with respect to our reflections past the initial 50 milliseconds or so.

So far we have looked the full spectrum RT. We know however that the low frequency modes likely are not following our rules. Let’s ask REW to show us RT60 for an octave of frequencies centered at 63 Hz:

We see a pretty chewed up line now since the reflection energy clearly is not random. We can try to fit a line to this but obviously there are a lot of deviations which REW notes with “r” values in orange. This means that the computation of RT for lower frequencies is probably not accurate.

Climbing up to 500 Hz cleans up the graph significantly:

With a nice line fit, you see why in research we used RT60 times for 500 Hz or higher. Let’s see what happens if we keep going up in frequency:

Even better. Now we are ready to go to the RT60 tab since you know what the different parameters mean. Here I have selected only Topt:

This is really a duplicate of our previous display except that the REW is doing the work for us of changing the frequency bands and plotting them as single values. We can easily read the mid-frequency RT60 times of 0.8 seconds. As a way of reference, the predicted RT60 using the Sabine formula for the same room is 0.66 seconds. Measured RT60 is higher due to one of the factor I mentioned: the absorption of material in the room is less than the ideal used in Sabine computation.

What does it mean to you?
A lot of objections were raised saying RT60 measurement is useless/meaningless in small rooms. With the knowledge at hand of what it is and how it is measured in a real small room, let’s see if that is the case.

Imagine you are playing a movie and actor says the word “hat.” We have three distinct parts to that with different loudness levels: “h,” a” and “t.” Let’s say it takes one second to pronounce “hat.” That means each part of it roughly takes 1/3 of a second or ~0.3 seconds. In our sample room we had a reverberation time of 0.8 seconds. This means that the pronunciation of “h” lasts another 0.8 seconds after it stops. That 0.8 seconds then overlaps the rest of the word. That may make it harder to hear the softer (in level) parts of that word. We may think the person said “had” instead of "hat." If we shortened our reverberation time to say, 0.2 seconds, the chances of that happening becomes much smaller and intelligibility improves.

Note that you don’t want to go too far here. The reflections help make the direct sound louder and hence easier to understand. Think of trying to talk to someone far away outside. It takes more energy than being indoor, right? Now you see why we have a lower bound for RT 60 values. The early reflections can be useful and we want to preserve them. But have the later ones die fast.

So as you see, RT60 is not useless at all. If we know what we are doing and measuring, we can extract useful data from it in order to determine if our room is too naked/live or alternatively over treated and too dead. Yes, with experience you too can determine the same by eye. Ethan did in his garage. And I could too without him posting the recording. That doesn’t invalidate RT60. It just says that our ears and experience can show the same. Indeed, Sabine did all of his measurements using his ears just the same!

If someone wants to challenge all of this, I hope they post measurements and data demonstrating their case.

 

[amirm]

Too much travelling has gotten in the way of dealing with this last argument which is almost always quoted verbatim from Davis’ text:

In case it all needs to be laid out, Local pointed out (and I agreed) that RT60 is an invalid calculation in small rooms because RT60 is the measurement of the decay time of "a well-mixed reverberant sound field well beyond critical distance".

There are two parts to this: “well-mixed reverberant sound field” and “well beyond critical distance.” Let’s deal with the latter first.

Critical Distance
Critical distance is the distance from the source at which point the level of the direct sound matches the sum total of reflections. The direct sound is what comes to you directly from the speaker. The reflections are caused by the indirect sound bouncing off all the surfaces. Since reflections on the average have longer paths before they get to us, then their level drops more than the direct sound. At the same time, if we keep moving away from our direct sound, that too drops. At some point the sound pressure level from both of these become equal. That is the critical distance denoted by “Dc” not be confused with direct current which we write as DC.

Well Mixed Reverberant Sound Field
There was an assumption in the above explanation: the level of reflections stayed the same even though we kept moving away from our source. For that to be true, the reflections need to be all around us and not location specific. If that is the case, then we can never get farther from them and hence their level will remain constant. That is what Davis means by “well mixed reverberant field.” Another name for it is diffused field. Another is randomness of reflections, modes, etc. All concepts that I have explained before.

Computing Critical Distance
There are a number of formulas for computing Critical Distance. For now, let’s go with one of its simpler form: 0.03121*SQRT(Q*V/RT60). V is the familiar volume of the space. RT60 you already know and is the reverberation of time of the room. Q is the measure of directivity of the source. If the source is omnidirectional, Q=1 and can be eliminated leading to the more simplified version of this formula without it. Higher values connote a more directional source. For example the human voice has directivity of about 2 as your face somewhat projects the energy away from your face.

Our interest here is speakers which have frequency dependent directivity. While designs vary, in general speakers get directional at high frequencies. Conversely at low frequencies they tend to have broader radiation pattern. Determining the actual directivity of a speaker can be difficult if the manufacturer does not provide it. That said, our interest her as with RT60 is the mid frequencies starting at 500 Hz. At 500 Hz, speakers tend to have pretty wide dispersion. I am going to pick a rough (effective) estimate of 150 degrees horizontal and 100 degrees vertical. That gives us a directivity of 3.7. So while higher than a human voice, it is not hugely different. This is why a human can replace the role of a speaker in some ad-hoc room testing.

For my 21x11x9 dedicated theater, using a Q of 3.7 gets us a critical distance of 3.2 feet. For a human talker with a Q of 2, the distance is a short 2.4 feet. I don’t recall my exact measurement distance for that room but it was in the order of 10 feet. This puts the measurement mic at 3 times the critical distance for the speaker. This may not be “well past Dc” but sure is in the neighborhood since the direct sound is dropping at 6 dB/doubling of distance resulting in 9 dB total drop below the reflected sound. Its contribution to total sound arriving at our ear/mic is pretty low then. If that were not low enough, we have plenty more distance to step back into since our room length is 21 feet (~7X critical distance). That said, this computation may not be correct. See the next section.

Plugging in Ethan’s garage and using a Q of 2 because he was making some of those noises with his mouth, and an estimated RT time of 2 seconds, his critical distance is just 1.9 feet. With a room length of 24 feet, he could go way, way past the critical source if he wanted. So the notion that we have to be “well past Dc” in a classic sense is satisfied here.

Limitations of Critical Distance Computation
So far, we have lived in an idealized space. Real spaces, large or small, don’t work that way. As with the Sabine RT60 equation, as soon as you add any absorption to the room, you lose your diffusivity. Sound hitting those surfaces does not reflect back and even if it did, it would not do so in a flat way with frequency. Put audience in a large concert hall for example and all of a sudden the entire floor area acts like a giant absorber so even though that room is “large” it may have lost its diffusivity/randomness just the same. So technically speaking, rooms big or small if they have absorption in them, they are bound to show error in their Critical Distance computation as well as their RT60. Question then becomes how big this error is in practice.

How about Expert Quotes?
Both Dr. Toole and Davis were quoted as saying we do have a “small room” problem when it comes to these measures. Conclusion was therefore reached that we could never apply these measures to any “small” rooms, including such spaces as Ethan’s garage. Let’s review what they really said so that can see if that was right.

Don Davis’ Sound System Engineering
We were told to read chapter 7 of this book. If we do, we see this explanation:

Our work with the TEF analysis process has confirmed that the simple equations that work so well in “live” rooms should simply not be applied in any form in small dead rooms….In very absorptive rooms, the sound dies away in a few reflections, and the statistical basis of the formulas is weakened. In recent studies done with time energy frequency analysis, typical meeting rooms in hotels have been found in some cases, RT60 = 0.5 s, to develop no reverberant sound field, whereas in others, RT60 = 0.7s, a field barely appears. Our experience with time-energy-frequency measurements causes us to state unequivocally that recording studio control rooms are not proper subjects for use of classic statistical equations.

The emphasis that I have added should already tell you what is wrong with the conclusions drawn. But let’s spell them out:

1. It is absolutely clear that Davis is railing against the simplified formulas, not measurements. I hope you remember the distinction from my last post. Measurements are immune to many of the errors of simple Sabine equations since they measure the actual sound level at our seating position. For example, lack of diffusivity means that we don’t have the same sound level in every place in the room. But we don’t care about every location. We care about where we sit and the mic picks up the sound level there. That measurement does not rely on us having the same reading elsewhere. Nor does it care about the speaker spec. If we change the Q of the speaker, it will be reflected in what the mic “sees” in our location of interest. That is very different than us plugging the wrong Q into the critical distance formula.

2. There is no better proof of #1 than seeing Davis use RT60 *measurement* himself! And what does he use it for? To determine whether a room is reverberant or not. Well, that is precisely the use we have for RT60. The fact that at 0.5 RT60 time the room loses a lot of its diffusivity/randomness is understood and indeed is the reason we want to add additional absorption: to get rid of excess reverberation/late reflections.

3. Davis acknowledges that reverberant field starts to appear at RT of 0.7. I gave a simple example of my room which with carpet and two rows of seating had similar RT. Clearly then it is possible to have residential spaces that cross the threshold into having a reverberant field. Ethan’s garage likely has RT60 times of ~2 seconds. If 0.7 is onset of the reverberant field, then surely 2.0 seconds is way into it being there.

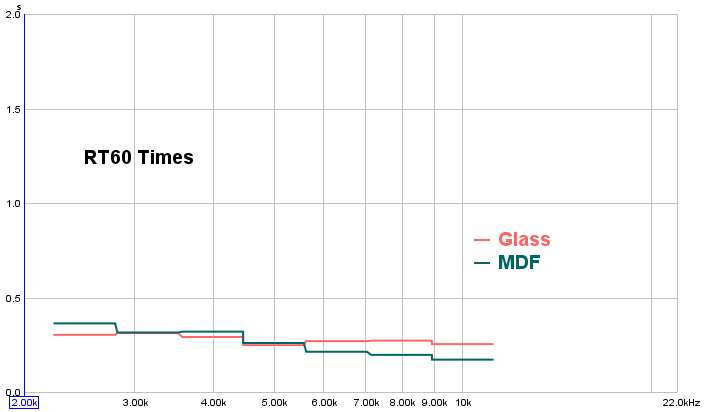
4. Davis is using “frequency blind” RT60. As I showed in my last post, we can look at specific mid-frequencies and eliminate the low frequencies. In doing so, we actually arrive at more accurate metric then he did because it does not vary as much due to changes in location. This is useful in home theater design where we want to know how every seat measures, rather than just one.

5. Davis reserves the strongest words against use of Sabine formula for “recording studio control rooms.” As a rule, folks in that space, especially in the 1980s when he wrote that book, were strong believers in eliminating first reflections. Popular concept such as Live-end, Dead-end (LEDE) for example attempted to eliminate the reflections from the front of the room. If you substantially reduce the power of the first reflections, the rest do die quickly as he states. So it is not surprising that Davis did not find a reverberant field in such rooms. And how did he confirm that? With measurement: “our experience with time-energy-frequency measurements…”

Summarizing, it is abundantly clear that Davis is admonishing us from using simplified formulas to characterize small dead spaces. That does not apply to Ethan’s garage that started this argument. His room is anything but dead and he used his ear which is very different than using a formula. Nothing is being said about small rooms automatically invalidating RT60 measurements since he himself uses this measurement.

Dr. Toole
As with Davis’ text, critical parts of Dr. Toole’s writing were left out which shine a proper light on this topic. From his book, Sound Reproduction:

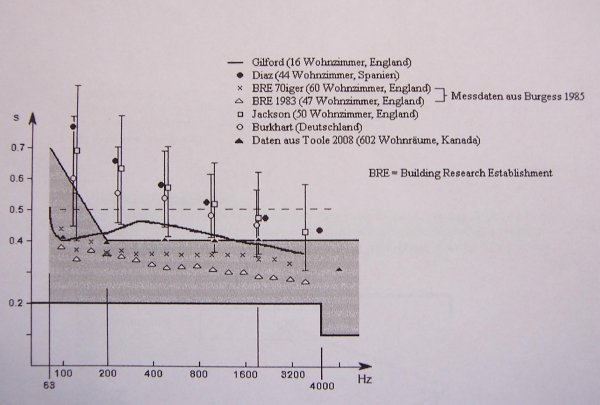
None of them [sample of rooms examined in the referenced paper] exhibited isotropic distributions at the measurement locations. Strong directional features were associated with early reflections. Small meeting rooms and a videoconferencing room with reverberation times of 0.36–0.4 s, like listening rooms, had anisotropy indices and directional diffusion measures that fell roughly halfway between anechoic and reverberant conditions. Moreover, the values changed with time, with later sound showing increased anisotropy and even changing orientation in the room according to the surfaces that were more reflective (Fig. 5).”

Once again we see that RT60 measurement is not only believed, but actually used to characterize how reverberant the room was – precisely what we use it for. The fact that the room was not isotropic (sound arriving from every location equally as I explained for Critical Distance) did not render RT60 invalid. It would have rendered the formulas less accurate.

Furthermore, as I have explained, it is very true that once you add absorption to get the RT down to recommended level, in this case in the 0.4 range, then your room stops having substantial amount of reverberation/diffused field. But our rooms if they are empty, and Ethan’s garage certainly, don’t start there. Again, my room even with inclusion of carpet and chairs which added substantial amount of absorption due to their large areas still had RT times 0.7 And Ethan's garage much more. These are not not the scenario that Dr. Toole is covering above.

If we wanted to reach into literature, we could find countless references (including some peer reviewed ones) where RT60 is used. Here is an example referenced by Dr. Toole in his book: “Acoustical Measurements in Some Canadian Homes,” Canadian Acoustics, Bradley, J.S. (1986):

The mean measured receiving room reverberation times and a one standard deviation range are given in Figure 7. In all 1/3 octave bands the mean reverberation time was close to 0.4 seconds with a standard deviation of just under 0.1 seconds.

The above was the mean and deviation of 602 homes surveyed in Canada. Clearly then RT60 is being used to characterize “home” and “small” listening spaces.

BTW times have changed relative to when that study came out. Trend these days is toward a lot more hard surfaces including the key elimination of carpets in favor of hardwood floors. If your room falls in that category, then it is likely to be very “live” with RT60s confirming the same. Here is another personal example in the form of our living room:

RT60 is a whopping 1.7 seconds at mid frequencies. Sadly it sounds that way too. Speech intelligibility is very poor. Get past 6 or 7 feet and it starts to get hard to understand someone over say the background level of TV playing. And forget about understanding them from adjacent room. The room is very bare as this is our vacation house and we have not put much furnishing in it. If we did, we would have this type of experience outlined in Dr. Toole’s book:

Once again we see RT60 measurements being used by the very experts who were portrayed as telling us to not use them. Notice how adding furnishings which act as absorbers, the RT60 time nicely tracked with them. If RT60 generates meaningless data, it should have shown random changes. It did not. More absorption = Smaller RT60 times, precisely what the Sabine formula predicted. Mind you, the changes may not be proportional to exact specs for those “absorbers” but it did track them generally. We don’t need more precision than that.

What does it mean to you
There is little need to measure the critical distance in home listening spaces. There is however usefulness in using RT60 to determine if your room is too live or too dead. You have seen examples of this from the experts quoted and if it is good enough for them, it certainly is good enough for the rest of us .

Now, if you don’t have measurement tools that is OK. You can do as Ethan did in his garage and use your ears and convenient noise source. Have someone stand where your speakers are and clap their hands. If you hear a long “reverb trail” , then your room is too live and needs general absorption. That can come in the form of furnishings such as carpets, drapes, etc. or purpose built absorbers. It matters not where you put them since the aim is to reduce general reverberations, not specific early/strong reflections. Note that you don’t want to clap yourself as that would mean the speaker is in the same spot as you which is not how it is in real life.

So as you see, no matter which way we approach the topic, the results are the same: RT60 measurement works and is a useful tool to estimate the amount of late reflections in the room. While the formula version can generate less accurate results (in both small and large rooms), it too can be useful if for example you have not yet built your room and want to estimate how much total absorption you should have. Dr. Toole gives an example of this in his book. Hence the comment: "In the meantime, the simple Sabine formula provides estimates that are adequate for our purposes in small listening rooms."

 

[amirm]

I was going to post this a few posts back. Well maybe more like about a hundred posts back. Anyway, I have a feeling my room is too dead. I have Linacoustic on the walls up to a height of 47" and the back wall is completely covered with Linacoustic from floor to ceiling. I also have a tiny room (about 9' x 12.5'). I have put in 4 DIY bass traps that are 4"x24"x24" at the 4 corners on the floor.

Here is my Topt as measured by REW with the front L and R speakers:

I contacted Greg offline and he kindly provided some additional detail on the dimensions of the room and specifics of the material on the surfaces. I thought it would be a useful exercise to walk through the math and science we have discussed and see what that gets us.

Recall the Sabine formula: RT60 = K * V / Sa. Let's see if we can compute RT60 using this and compare it to the actual measurement posted by Greg. V = 785 for Greg's room. K is 0.049 for Imperial system (feet). Sa is the surface area times the absorption coefficient(alpha). In the case of Greg's room, he used dissimilar material so we have more than one alpha. There is an easy solution for that. We can simply multiple the surface area for each type by its corresponding alpha and then sum them up all up. That gets us the total Sa which we can then use in this formula.

Turns out Greg's room is not a simple rectangle. So I had to do some rough estimation of actual surface areas. The right numbers are probably somewhat different than these but I think they are close enough to run with them. He has three types of material in the room:

1. Carpet on the floor. Coefficient of absorption for household items is hard to come by but we have some data that points to it being around 0.5 at 500 Hz, our frequency of interest for RT60.

2. Linacoustic. Greg used 1 inch thickness which the manufacturer shows to have 0.6 absorption coefficient at 500 Hz.

3. Bass Traps he built. I don't have an exact number for this but the material is thick enough that I used 1.0 for 500 Hz (i.e. total absorption).

Now let's multiple the surface area times the above alpha coefficient for each part of his room:

Front Wall 33.6
Back Wall 39.9
Left and Right Walls 67.2
Floor 56.25
Ceiling 48
Traps 16
Soffit 12.6

Total = 273 Sabines

Putting that in the sabine formula, we get an RT60 at 500 Hz of 0.14. Eyeballing the actual measurement above, I see values in the 0.11 to 0.12 in the graph at mid-frequencies. We see that this 120 year old formula is proving remarkably accurate! It certainly good enough to tell us if our room is on the dead side (at or below 0.2) or live side (> 0.5). The fact that Greg thinks his room is dead provides additional validity to the computation and measurements confirming the same.

As I noted and quoted Dr. Toole, for the purposes of estimating how much absorption we need to have in a room before building it, the formula can be quite useful. It is also useful for Greg to look at the above break down and decide how much impact each can have if he shrunk their amounts. The actual difference there may be more in error because the room is highly dead right now but is enough to make some reasonable decisions. For example we can see that the traps are not contributing much due to their much smaller surface area.

Useless/Meaningless data? I think not .

 

[amirm]

Ethan's 37.75" by 22.5" by 14.5" empty, glass-lined box has an RT60 of approximately 0.3, which according to you is just about right, or perhaps slightly on the dead side. Is Ethan's RT60 measurement providing useful information?

Thank you for your question. Before I answer it, I hope you join me in commending Ethan for taking the time to set up such elaborate fixtures to test the notions posted on the Internet. I always give him kudos for having an inquisitive mind that wants to prove to himself that his understanding of the science is correct.

Back to your question, the breadcrumbs are in this thread to answer that. Let’s review them again as a refresher.

The box, and let’s be clear that it is a small one given those dimension in inches, has very small amount of volume:

By my math it is only 6.5 cubic feet. In one of my earlier posts (http://www.avsforum.com/t/1453370/d...oticeable-audible-difference/90#post_23008908) I talked about the Schroeder transition frequency. This is the frequency below which the modes are too separate making our room response more location specific. For our typical home listening spaces, that frequency is usually in the 200 to 300 Hz region so you almost don’t need to do the math there. This little box however is far smaller than a real room so let’s do the math for it:

Fc = 11885*SQRT(RT60/V); let’s plug 6.5 in there for Volume and stated 0.3 RT60 time. That gives us the Transition Frequency of 2,535 Hz. Alas, the Schroeder’s formula was arrived at empirically (working backwards from measurements) and never tested against such a little box. The way we compensate for that is to instead think of this as somewhere in the middle of a much wider range of frequencies (much like I did in my “200 to 300 Hz” statement above). I don’t have direct data on how wide we need to make it in this small space but I think it is safe to say that the transition region could easily range from 2000 Hz to 3000 Hz. Let’s park this for a moment.

The idea behind a target reverberation time is to balance two needs: 1) speech intelligibility which is important in both movies (dialog) and music (singer) and 2) nice feeling of space we get from room reverberations. These two factors kind of fight against each other to some extent as ideally we like to have both. That is why we have a range specified of 0.2 to 0.5 rather than a single number. This range however is not computed but based on industry's collective experience of what the value should be based on countless in field experiences in home listening spaces. That experience has been formed from typical home spaces, not a small box like this or an auditorium. The latter actually has its own set of recommended RT numbers which are larger than this. There has been no interest in characterizing how good speech sounds in such a little box so hence, there is not a recommended range for it.

Ignoring the above fact for a moment, we can walk through the analysis anyway and see where that gets us. We have an RT60 time of 0.3. Numerically 0.3 is in that range but there is a problem: our transition frequency has shot up by a factor or 10 over a typical room. Recall that I said in the research and industry our target frequency of interest is 500 Hz although that is often stretched to 1K to 2K Hz. One of the reasons for this was the fact that we would be free of the modal issues (large response variations) below transition frequencies of 200 to 300 Hz of our rooms. We immediately see a problem here. We can’t use RT60 @ 500 Hz since a) Ethan did not test that frequency and b) it would be below the transition frequency.

You might be asking why we don’t look at higher frequencies since they would be above transition range for this box and we do have the data for that. That does not work either. Look at the spectrum of speech in this sample spectrogram as the person pronounces “Rice University:”

This is a nice visualization of the problem with speech intelligibility and late reflections. It is clear from the time graph at the bottom that the intensity of speech changes every 0.2 seconds or so in English language (it may be faster or slower in other languages). The larger colorful graph shows the frequency spectrum at any moment and color coding of the energy/strength. Red means very strong, blue means very week. It is pretty clear that the bulk of vocal energy is below 2,000 Hz. It therefore matters not what higher frequencies are doing as they don’t have a lot of energy at the start. Evaluating RT60 times well into Khz region therefore is not material to this analysis. It is like trying to figure out engine idling problems in a car with a tachometer that starts at 2000 RPM.

Fortunately there are no applications for dollhouse sized home theaters or listening spaces so there will be no riots in the streets that we can’t perform such an analysis.

BTW, there is a small listening space of high interest to us: cars. Their smaller volume means that the modal region climbs up to 500 Hz or even higher. Getting smooth response is hard in our homes below 100 Hz. Now imaging that problem multiplied and moves up in frequency, now impinging on low range of even voices! Inversely, as I mentioned before, larger performance spaces have much lower transition frequencies usually below 20 Hz, which eliminates the modal concerns altogether. These are the factors that are important as we look at “small” and “large room” acoustics, not some worry about RT60 measurement being wrong.

Back to Ethan’s box and experiment, he was simply comparing two surface materials against each other: MDF vs. glass. We know glass is smoother than MDF resulting in theoretically less absorption in higher frequencies. Less absorption means higher reverberation time (what doesn’t get absorbed, gets reflected). Since the measured RT60 time tracked this difference correctly, we have yet another validation point that this measure is accurate! Here is his RT measurement:

If RT60 measurements were useless then we should have arrived at random data and not have experiment after experiment, showing the correlation with amount of absorption introduced in the space.

BTW, the whole argument is circular anyway. You can’t prove RT60 measurement is meaningless using RT60 measurement itself! You have to have an independent metric that is believed to be true that shows RT60 to be wrong. This is how we showed error in the *computed* RT60. We trusted RT60 and showed that the formula must be in error.

 

[amirm]

So you're talking about syllables, I guess, and saying that intelligibility will sink to a minimum when an echo of the last syllable is heard at the same time as the current syllable? Sounds plausible, but on the other hand, you know, humans are probably well adapted to understanding each other in reverberant spaces. So maybe not.

You definitely have the right concept. It is just that it applies to early reflections (less than 0.1 seconds), not late. Early reflections “fuse” together with the direct sound to help increase the total power of the source, hence resulting in better effective signal level/intelligibility. Late reflections are not helpful in this regard. The brain stops accumulating such data after certain amount of time. If it did not do that, then it would not be able to hear clearly separate audible events. See the graph in my first post in this thread that shows this phenomena and levels/distances (i.e. delay) where it occurs: http://www.avsforum.com/t/1453370/d...oticeable-audible-difference/30#post_22977312

Here are some quick references:

Journal of ASA paper: Prediction of the influence of reverberation on binaural speech intelligibility in noise and in quiet

"…that reflections may be useful for the listener if they arrive within a certain time interval te after the direct sound, but disturb intelligibility if they arrive later (for details, see Sec. II B 3 or Bradley, 1986; Bradley et al., 2003; Bradley, 1998; ISO, 2009). These measures are widely applied in room acoustics, although the optimum limit (te) for the useful part in the range of about 50 to 100 ms may differ between studies."

Here is a useful graph from the referenced Bradley paper:

This graph is for larger spaces and hence the elevated RT values than what we are talking about in our home spaces. Still, you see that the shorter the RT time, the better the speech comprehension. An interesting observation from that data is that at some point, increasing the volume of the source no longer helps to improve audibility! The likely reason for that is that increasing the source volume also increases the level of late reflections so the net gain becomes zero (right side of the curves).

I should note that there is a lot of food fights in the literature on how proportional the intelligibility is to the numerical value of RT60 and other similar formulas. So don't go by this data religiously. We don't care what happens if you go from 0.31 RT to .037. We care that there is difference between that and say, 0.7.

This is less plausible. Consonants ordinarily do assimilate to following vowels, anyway, so I doubt that this "smearing" would affect intelligibility much.

Following vowel? The scenario was the other way around. RT60 must be too high in this forum.

Imagine if I said: “take the cat” vs. “take the cab.” The plosive consonants “t” and “b” are the only difference in those sentences and by definition are very quiet relative to vowel “a” preceding it. The late reflections from that vowel can impact the audibility there. Contextually you may be able to compensate between these two sentences but not always.

Here is a quick reference on that. Journal of AES [peer reviewed] paper: Audio Engineering and Psychoacoustics: Matching Signals to the Final Receiver, the Human Auditory System”

”Using the loudness exceeded in 10% of the time as an indication of the perceived loudness, it can be expected that the speech is 1.2 times louder in the room with 0.6-s reverberation time and about two times louder in the room with 2.5-s reverberation compared with the loudness produced in the free-field condition. This increment in loudness is often very helpful for the intelligibility of speech in rooms as long as the reverberation time does not produce temporal masking, which reduces the audibility of faint consonants appearing in sequence to loud vowels.”

Temporal masking means that an event earlier in time, steps on a later audible event which is what I explained.

This why we like to have certain amount of general absorption in the room, but not too much as to eliminate the good benefit of them helping with intelligibility and feeling of spaciousness/being in a real space. It is a balancing act and hence the recommended range.

 

[microstrip]

Amir,

Thanks for summarizing this polemic subject - I followed parts of it in the avsforum, but I did not feel competent enough to ask questions.

Here at WBF I feel more comfortable. How do you interpret Toole in page 509 of his book when he seems to say that in small rooms "the key is not the number, but the sound" or writes "Feel free to add or take materials until it sounds "right", RT numbers are not highly predictable"?

I have taken many RT60 measurements in my room, and feel happy they are good, but always feel uneasy with these statements.

Just one technical detail. I have found that some people measure RT using the main speakers in the listening position, others put two speakers in the center of the room facing opposite directions to simulate an omnidirectional excitation. What is the best technique - or better still : how do you do proceed to measure RT60?

 

[steve williams]

In acoustics, home listening spaces are considered to be small. Some people take that and then use it to invalidate some of the classic measurements such as RT60 which shows how long reflections take to die down in a room. I have argued this point elsewhere with a lot of detail, showing that if we know what we are doing, we can measure and trust RT60. Ethan was the subject of one of those discussions and he had the good idea of distilling it to a short article. I thought it would be a good read for folks here:

http://www.realtraps.com/art_reverb.htm

I will also post copies of my posts from other forums on this topic.

Completely agree

 

[amirm]

Amir,

Thanks for summarizing this polemic subject - I followed parts of it in the avsforum, but I did not feel competent enough to ask questions.

My pleasure. This is one of the reasons we created WBF .

Here at WBF I feel more comfortable. How do you interpret Toole in page 509 of his book when he seems to say that in small rooms "the key is not the number, but the sound" or writes "Feel free to add or take materials until it sounds "right", RT numbers are not highly predictable"?

If you read my posts you see that one has to be very careful to measure the proper range of frequencies. The general guidelines for RT don't say that. As a result, one man's 0.4 may be another's 0.6. Additionally, there is a range there for a reason. Preferences vary based on person and type of content you listen to. So to find the right balance, he is rightly suggesting to use one's ears to determine the final target.

For me, this is a two-step process. Measure RT60 and if it is say, 1.0, then you don't need to do listening tests. Your room is too live. Likewise, if it is .1, then it is too dead. Fixing these doesn't require listening tests. But once you get it within the range of 0.2 to 0.5, then listening tests is useful to find tune your target.

I have taken many RT60 measurements in my room, and feel happy they are good, but always feel uneasy with these statements.

That is actually OK. This is not a precision instrument and in the degree of importance, is not that high relative to say, smooth bass response, and good speaker selection.

Just one technical detail. I have found that some people measure RT using the main speakers in the listening position, others put two speakers in the center of the room facing opposite directions to simulate an omnidirectional excitation. What is the best technique - or better still : how do you do proceed to measure RT60?

If you use 500 Hz as I do, your speaker will have pretty wide directivity. 500 Hz is also high enough to be outside of the very modal region of bass frequencies so the corner position of the speaker does not load it up too much. Best thing to do is turn on the Schroeder integral and see how well the estimated Topt line tracks it. If it tracks it well, then you have the right configuration.

Omnidirectional is important if you care about high frequencies because speakers get very directional there. I see no value in measuring RT time for high frequencies. It is outside of the vocal range.

 

[Ethan Winer]

Excellent Amir, this should be made a sticky.

--Ethan

 

[amirm]

Sticky it is....

 

[KlausR.]

Hi Amir,

allow me to comment on some points:

Computing the transition frequency as the above number is called, we get 352 Hz.

In his original paper Schroeder proposed a formula which results in a tenfold modal overlap: “It is assumed that the number of simultaneously excited, independent modes is large enough to yield a Gaussian distribution of sound pressure components. Practical experience has shown that the approximation of the Gaussian distribution is good for about 10 independent components of the same order of magnitude.”

“If the average separation of eigenfrequencies is small compared to the average half-width of the resonance response curves, then the separation of the maxima is given by the half-width since an increasing number of maxima, generated by the individual eigenfrequencies, are “screened out’.”

* Schroeder, “Statistical parameters of the frequency response curves of large rooms”, JAES 1987, p.299 (translation of original paper (in German) published in Acustica 1954)


In a later paper Schroeder changed that to a threefold modal overlap: “at least three resonances fall within the half-power bandwidth of one resonance”, which halfs the value of the frequency as compared to the initial equation.

* Schroeder, “The Schroeder frequency revisited”, JASA 1996, p.3240

However, it has been found that this transition between individual, well separated resonances to many overlapping modes occurs at frequencies well above the Schroeder frequency, so that the initial formula would better be in line with what has been observed, which would put the Schroeder frequency in the 400-600 Hz range, rather than the 200-300 Hz you mentioned.

Baskind et al., “Sound power radiated by sources in diffuse field”, AES paper 5146

However, Schroeder’s goal was to obtain statistical parameters of frequency response curves (i.e. the curves’ range of variation such as average rms respones fluctuation, average height of a maximum, mean separation of zeros) that are independent of the location of source and receiver, but only depend on RT60. Nothing about smoother response, sound quality, existance of a diffuse field etc.

It has, as you’ve pointed out yourself, been found that in small rooms (but also in large rooms) the sound field is not diffuse but directional:

* Meyer, „Definition and diffusion in rooms“, JASA 1954, p.630
* Gover et al., “Measurements of directional properties of reverberant sound fields in rooms using a spherical microphone array”, JASA 2004, p.2138

Since the field is not diffuse, concepts like the critical distance no longer apply:

* Toole, “Loudspeakers and rooms for sound reproduction – a scientific review”, JAES 2006, p.451

However, you then wrote:

Once again we see that RT60 measurement is not only believed, but actually used to characterize how reverberant the room was – precisely what we use it for

If that is to be understood that there is a link between RT60 and the degree of diffusivity, then the data would not totally support this:

From the Gover paper:

Meeting room
102 cbm, RT60 = 0.36 s
diffusion = 70%, anisotropy = 4 dB
different receiver position
diffusion = 59%, anisotropy = 7.8 dB

Videoconferencing room
181 cbm, RT60 = 0.4s
diffusion = 58%, anisotropy = 7.4 dB

Lecture theatre
875 cbm, RT60 = 0.6 s
diffusion 61%, anisotropy = 5.5 dB

The data of Meyer (rooms of 300 – 15,000 cbm) show a trend of diffusivity decreasing with increasing RT60. He also found that within the same room values vary from place to place. He further found that the location of absorbing material in the room had an effect on measured diffusivity.

... once you add absorption to get the RT down to recommended level, in this case in the 0.4 range ...

The only source that mentions research w.r.t reverberation times in listening rooms is

* Gilford, “The acoustic design of talk studios and listening rooms”, JAES 1979, p.17

where an investigation by the BBC is described on half a page. The result then was 0.4 seconds. I wonder on what research are based the figures one usually encounters in recommendations such as EBU 3276, or such as the range of 0.2 – 0.5 seconds you mention.

This range however is not computed but based on industry's collective experience of what the value should be based on countless in field experiences in home listening spaces. That experience has been formed from typical home spaces, not a small box like this or an auditorium.

There are a few papers where RT in actual homes has been measured. I’ve made a graph showing the EBU 3276 requirements and the data of those measurements. Some of the figures fit within the recommendation as per EBU, some don’t. None go as low as 0.2 seconds.




The question is, at what value does RT begin to disturb, is 0.2 in studio control rooms really necessary, has this ever been investigated?

So as you see, RT60 is not useless at all.

The problem I see is that a single figure doesn’t tell you a lot: a RT60 of two seconds in a concert hall will be fine, the same figure in your living will be a disaster. My room is 100 cbm with a calculated (Sabine equation) RT of about 0.4 seconds. If I go to half that volume, will 0.4 seconds be too much? If I go to twice that volume, will 0.4 seconds be to little? Does 0.4 seconds feel the same everywhere, regardless of room size?

Klaus

 

[andromedaaudio]

Big spaces :

I was in the amsterdam concertgebouw today , also quite a famous hall for its acoustics , beautifull interiour too with its balconies .
i asked also about the reveberation time , the big hall : 2,2 seconds full with people , 2,8 in the empty hall.
small hall 1 second and lastly the smaller choir room in the basement well below 1 second .

i asked also what made the hall acoustics famous :
according to them no concrete anywhere , its made of bricks and a poreus plaster cover and wood off course .
absorption: wool covert seats and a fair amount of wool curtens .
its not a deep hall , it has irregular shaped floor walls ceiling so that would act as diffusion.
and lastly the peoples clothes in it act as absorption as well

 

[KlausR.]

There is some info on the acoustics of the Concertgebouw, if interested in a copy drop me a mail.

http://asadl.org/jasa/resource/1/jasman/v109/i5/p2304_s2

http://asadl.org/jasa/resource/1/jasman/v106/i4/p2300_s4

http://asadl.org/jasa/resource/1/jasman/v89/i3/p1176_s1

http://asadl.org/jasa/resource/1/jasman/v88/i4/p1811_s1


Klaus

 

[andromedaaudio]

I d sure be interested i ve sent a mail , thanks

greetz hj

 

[Jose Almagro]

The question is, at what value does RT begin to disturb, is 0.2 in studio control rooms really necessary, has this ever been investigated?

I'm pretty sure you know a higher Tm than recommended can be nice. I think Bob Walker was the head of the group that developed the EBU 3276 and he designs studios with high Tm so we can keep 0,2 as a common recommendation, not a dogma.

 

[Sam Ash]

Measuring RT60 in Room EQ Wizard

If you use 500 Hz as I do, your speaker will have pretty wide directivity. 500 Hz is also high enough to be outside of the very modal region of bass frequencies so the corner position of the speaker does not load it up too much. Best thing to do is turn on the Schroeder integral and see how well the estimated Topt line tracks it. If it tracks it well, then you have the right configuration
.

Hi Amir, I'm new to the forum and have found your tutorials and explanations to be very useful and informative. Just needed you to clarify something: I have a 7.1 HT configuration for movies and music. For RT60, does one use a sweep going from 125hz to 2,000Hz ? Do these values have to be entered into REW before generating the sweep ? Furthermore, do you use just one of your front speakers for the RT60 ? i.e. - the left, center or right front speaker ?

 

[amirm]

Hi Amir, I'm new to the forum and have found your tutorials and explanations to be very useful and informative. Just needed you to clarify something: I have a 7.1 HT configuration for movies and music. For RT60, does one use a sweep going from 125hz to 2,000Hz ? Do these values have to be entered into REW before generating the sweep ? Furthermore, do you use just one of your front speakers for the RT60 ? i.e. - the left, center or right front speaker ?

Hi Sam. Thanks for the kind words.

With REW you do one sweep and it generates all the other measurements from that one. Make it full bandwidth (20 to 20000) for completeness. But with respect to RT60 pay attention to the value shown for 500 Hz. It is this value for which we have the rules of thumb for RT60:

As to which speaker to use, you can use any of them as this is a measure of overall reverberations in the room. For the sake of specificity, use the center channel since the above rules of thumb are for speech intelligibility and that is where the voices are typically in movies.